Circle sector - perimeter and area
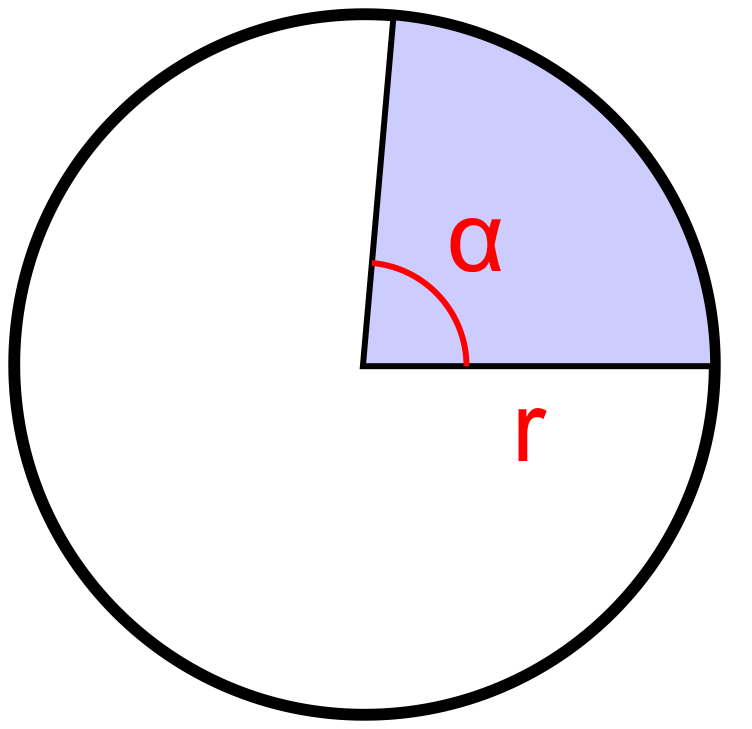
C = 2πr. α / 360°
A = πr2. α / 360°
A = area
P = perimeter
α = angle
r = radius
WHAT IS IT ?
A circle sector is a region of a circle enclosed by two radii and the corresponding arc. The radii are straight lines connecting the center of the circle to two points on the perimeter, and the arc is the part of the circle's perimeter that lies between those two points. Circle sectors can be classified into two types:
1. **Minor Sector**: The smaller area enclosed by the two radii and the arc.
2. **Major Sector**: The larger area enclosed by the two radii and the arc.
The properties of a circle sector include the central angle, which is the angle between the two radii, and the arc length, which is the distance along the curved boundary. Circle sectors are used in various applications in mathematics, engineering, and design, especially in calculating areas and lengths related to circular shapes.
CALCULATION:
EXAMPLE:
The radius of the circular sector is, for example, 6 cm and the sector angle α is 45°.
Thus,
r = 6,
α = 45.
Circumference of the circular sector:
We use the formula
O = 2πr · α / 360°
thus
O = (2*3.14*6*45) / 360
O = 4.71 cm
Area of the circular sector:
We use the formula
A = πr² · α / 360°
thus
A = (3.14*6*6*45) / 360
A = 14.14 cm²
